Rook Polynomials: A Straight-Forward Problem – Feature Column
Por um escritor misterioso
Descrição
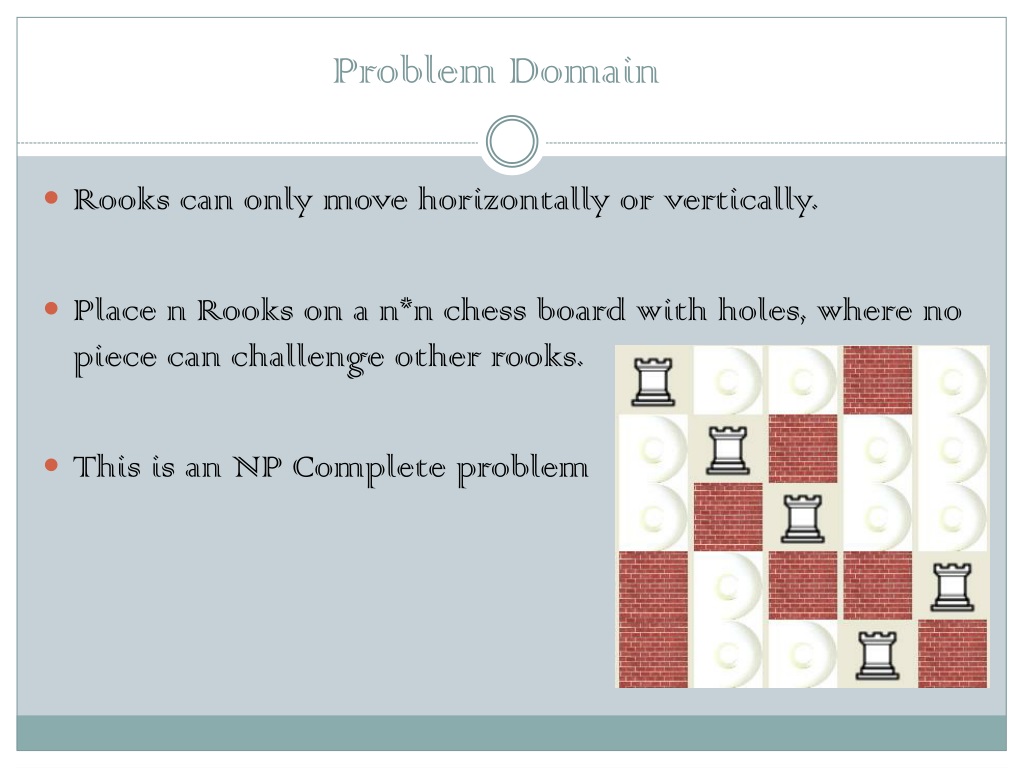
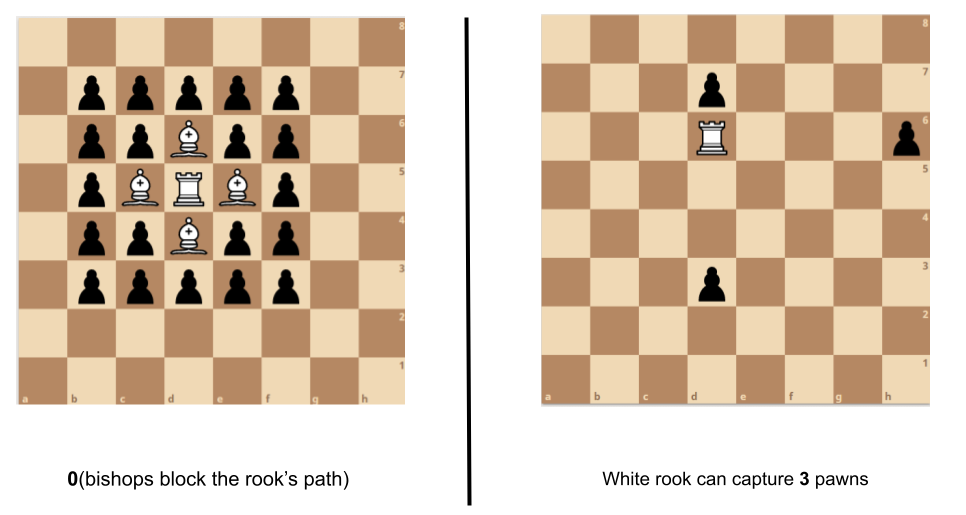
Rook Polynomials: A Straight-Forward Problem For an integer $k$, is it possible to place $k$ rooks on a chess board so that no piece sits on the same row or column as any others? We wouldn’t want them stepping on each others’ toes. Tamsyn Morrill Trine University In the game of chess, each of the…

combinatorics - Use of rook polynomials - Mathematics Stack Exchange

The Kalman Filter. Helping Chickens Cross the Road. – Feature

Polinomios de Rook, PDF, Mathematics
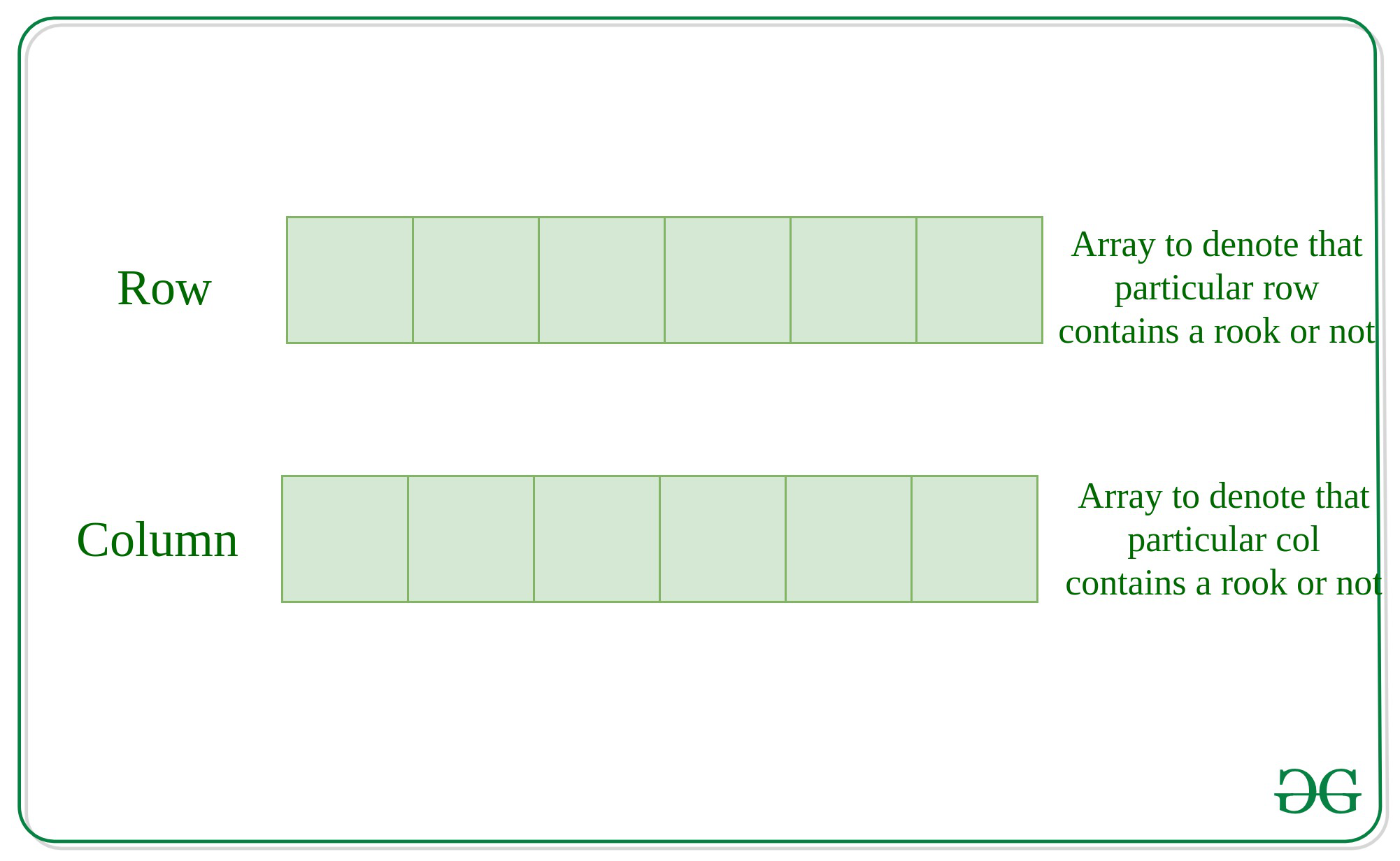
Find position of non-attacking Rooks in lexicographic order that

combinatorics - Use of rook polynomials - Mathematics Stack Exchange
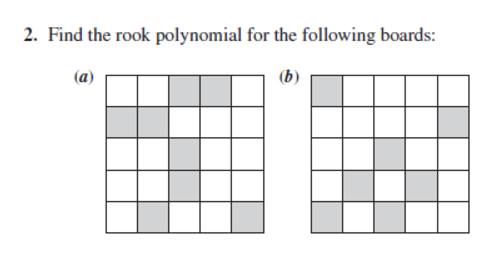
Solved Find the rook polynomial for the following boards

PDF) On selecting exactly k columns from a matrix
There is a 10 × 10 table (”chessboard”). Two players make moves in
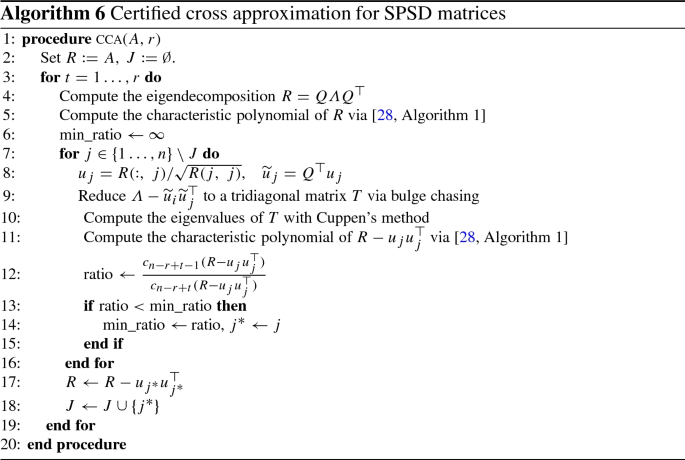
Some algorithms for maximum volume and cross approximation of

The chi-square matrix constructed from a set of 50 random solutions

Polinomios de Rook, PDF, Mathematics

DSU/Union Find Fundamentals